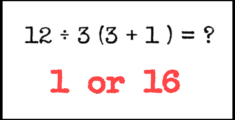Math problems designed for kids are supposed to be easy, right? But every once in a while, a simple question for elementary school students ends up baffling adults. That’s exactly what happened with this math problem that’s gone viral online.

What makes it so confusing? Adults often overthink things, trying to find hidden tricks or overcomplicating the process. Teaching methods have also changed over the years, so what seems straightforward to kids today might feel unfamiliar to someone who learned math decades ago.

This particular question has sparked debates at offices, dinner tables, and on social media. People are arguing over the “correct” answer, and in some cases, the discussion got so heated that mathematicians were called in to settle it!

Why has this problem caused so much buzz? Maybe it’s the challenge of proving you’re right. Or maybe it’s just fun to revisit simple math and see if you’ve still got it.

Now it’s your turn. Can you solve the math problem that has everyone talking?

Were you a math whiz back in elementary school?

I can’t say I was. However, as an adult, I feel confident I could solve a simple math problem meant for kids.

But here’s the catch—this particular math question has taken the internet by storm. People everywhere are debating the correct answer, turning it into a hot topic at workplaces and dinner tables across the globe.

Can You Solve the Kids’ Math Problem That Went Viral Online?

The argument over the right answer to this math question got so heated in some offices that people even asked mathematicians to figure it out.

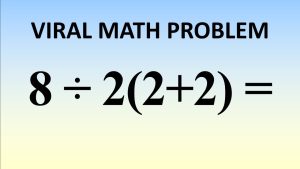
Can You Solve It Correctly?

A Simple Kids’ Math Problem With a Controversial Solution Some people say the answer is 16, while others insist it’s 1.

But how can a math problem have two different answers? Isn’t math supposed to be straightforward, with one right answer and one wrong answer? Unlike other subjects, math is meant to leave no room for interpretation—so why the confusion here?

This particular problem is a bit tricky and depends on how well you remember the rules of elementary school math. So, why does this simple children’s math problem lead to two completely different answers? The solution lies in something called the order of operations.

The order of operations is the set of rules that determines the sequence in which parts of a math equation must be solved. To help remember this sequence, children are often taught the abbreviations BEDMAS or PEMDAS, depending on where they live. These stand for:
The correct answer is and will always be 16, regardless of whether you use PEMDAS or BEDMAS.

That’s the Key

The important thing to remember is that the letters D and M in BEDMAS or PEMDAS stand for division OR multiplication (or multiplication OR division). This means you solve them in the order they appear from left to right, not based on how they’re listed in the acronym.

In this problem, the first step is to solve what’s inside the parentheses: (2 + 2) = 4.

After that, the equation simplifies to: 8 ÷ 2 (4).

Note that multiplication is actually indicated by a number next to the number in parentheses. The equation is now 8 ÷ 2 × 4.

Now that division and multiplication are the only remaining tasks, just calculate the equation from left to right, starting with division:

8 ÷ 2×4 = 4×4 = 16

The debate over this children’s math problem highlights just how easily confusion can arise, even with simple equations. It underscores the importance of understanding the order of operations, which is key to solving mathematical expressions correctly. The fact that people arrived at two different answers—16 and 1—shows why it’s so important to review and clarify these rules, especially for those who may not have practiced them in years.

In the end, the correct answer to the problem is 16, when the order of operations is applied correctly. By following the PEMDAS or BEDMAS rules, we solve the parentheses first, then perform division and multiplication in the order they appear from left to right. This basic understanding is crucial, not just for solving this problem, but also for handling more complex equations in the future.

If you are still not clear about the sequence of procedures, watch the video below.

As adults, we may sometimes overlook these foundational principles, but revisiting them can sharpen our problem-solving skills and help us communicate better with younger learners. Engaging with math in this way encourages a deeper understanding and appreciation of the subject, making it both a vital skill and an enjoyable challenge. Whether tackling children’s problems or advanced equations, mastering math’s basics is essential.